Local nonsatiation

The property of local nonsatiation of consumer preferences states that for any bundle of goods there is always another bundle of goods arbitrarily close that is preferred to it.^[1]

Formally if X is the consumption set, then for any 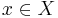$x \in X$ and every 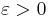$\varepsilon>0$ , there exists a 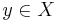$y \in X$ such that 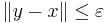$\| y-x \| \leq \varepsilon$ and $y$ is preferred to $x$ .

Several things to note are:

1. Local nonsatiation is implied by monotonicity of preferences, but not vice versa. Hence it is a weaker condition.

2. There is no requirement that the preferred bundle y contain more of any good - hence, some goods can be "bads" and preferences can be non-monotone.

3. It rules out the extreme case where all goods are "bads", since then the point x = 0 would be a bliss point.

4. The consumption set must be either unbounded or open (in other words, it cannot be compact). If it were compact it would necessarily have a bliss point, which local nonsatiation rules out.

Notes

^ Microeconomic Theory, by A. Mas-Colell, et al. ISBN 0195073401